Part 1 - Measuring the Density of Metal Cylinders
Purpose - We will be introduced to propagating uncertainties in the measurements we take for our data which leads to uncertainty in the final result. This simply means that we are going to find a range of values that will be with-in the accepted value. For instance, if we take a pen and weigh it on a scale. The scale is a cheap one so the range of uncertainty on the weight of the pen will be +/- the value on the display of the scale.
Purpose - We will be introduced to propagating uncertainties in the measurements we take for our data which leads to uncertainty in the final result. This simply means that we are going to find a range of values that will be with-in the accepted value. For instance, if we take a pen and weigh it on a scale. The scale is a cheap one so the range of uncertainty on the weight of the pen will be +/- the value on the display of the scale.
Step 1 - For this lab we are given three different size metal cylinders. With a scale and caliper we are to measure the weight, diameter and length of these three objects.

As you can see highlighted we have the value the scale displays, in blue. Something to note is in red. That is the uncertainty value +/- 0.1 grams from the measuring tool. Which we will need later.
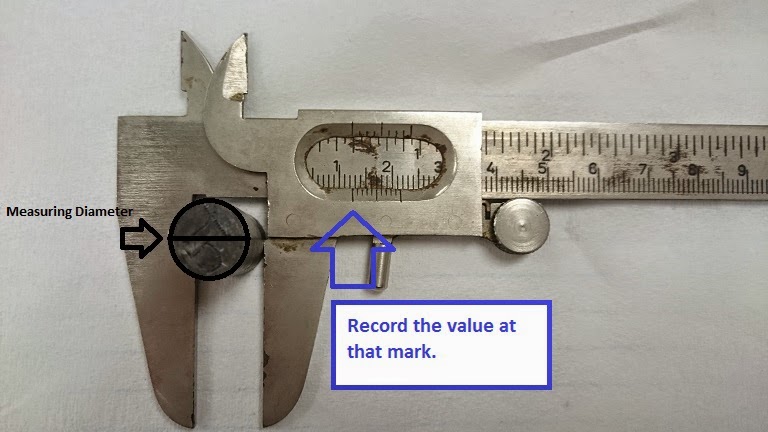
Image Below: Here we have a caliper that will measure the length and diameter of the metal cylinder. As you can see it is pretty difficult to read the value. Notice the blue arrow points to the tick mark that we will use to record the data. If you look closely the tick mark is between the 1.2 cm and 1.3 cm. To record our data we use an educated assumption that the value the caliper is providing us with, is a diameter of 1.24 cm.
Image Below: This is our data table we collected from measuring all three metal cylinders with the tools mentioned above. Note that with the size difference, some how the masses are identical to the tenth decimal place.
If we think about the different densities of metal in the world. We can assume that lead is denser than iron and iron denser than aluminum. Given our type of metal cylinders we know that we can find an actual value that we can compare with our record data. Doing so will brighten our insight on how uncertainties in each measurements will effect our final recorded value.
Note that height, diameter and mass we recorded has an uncertainty. As we find densities for each metal cylinders they too will have an uncertainty from the measurements written.
Step 2 - The data we record will assist in determining the densities of these three objects.
Image on right explains the density formula.
If you notice the data we recorded is missing volume. Therefore, we are going to use another formula that will give us our volume.
Image on right explains the density formula.
If you notice the data we recorded is missing volume. Therefore, we are going to use another formula that will give us our volume.
Image below gives us an understanding on how we will find our volume.
pi is 3.14...
r is radius which is half of a diameter
h is height
r is radius which is half of a diameter
h is height
If we combine the volume formula into the density formula we will obtain a means to calculate our density with the information we have.
Step 3 - Propagating the uncertainties of each measurement.
To propagate an uncertainty in the measurements we will use a calculus technique called partial derivatives. It follows some of the same principals as deriving equations. Instead of traditionally taking a number and variable and deriving. We will use our formula of density that we substituted the volume of a cylinder into.
Note we have three variables; m for mass, d for diameter, and h for height.
We will take each variable and partially derive each of them. Each variable we partial derive will also be multiplied by the uncertainty from the measuring tool. We accomplish partial derivative by taking which ever variable we will partial derive, derive that and treat everything else as constants.
For example, if our mass was the variable we are partially deriving. The diameter and height variables would act as a constant and as I would say "move to the front of the bus." No need to do that power stuff like bring the n down and subtracting n by one afterwards. That only applies to our mass variable.
Same goes if diameter was our variable we are partially deriving. Mass and height would be the constants.
Note we have three variables; m for mass, d for diameter, and h for height.
We will take each variable and partially derive each of them. Each variable we partial derive will also be multiplied by the uncertainty from the measuring tool. We accomplish partial derivative by taking which ever variable we will partial derive, derive that and treat everything else as constants.
For example, if our mass was the variable we are partially deriving. The diameter and height variables would act as a constant and as I would say "move to the front of the bus." No need to do that power stuff like bring the n down and subtracting n by one afterwards. That only applies to our mass variable.
Same goes if diameter was our variable we are partially deriving. Mass and height would be the constants.
If height is the variable were partially deriving. Mass and diameter would be constants.
Note that the funny looking d is the symbol to describe partial derivatives.
Once we get our values from partially deriving each variable we will absolute each value and sum them all up. That value then becomes our uncertainty for density of which ever metal cylinder is plugged in.
Below Image: This is a sample of how we calculated one of our metal cylinders.
Our calculated results
Density for lead and uncertainty: 11.55 g·cm−3 +/- 0.2808 g·cm−3
Density for iron and uncertainty: 6.72 g·cm−3 +/- 0.3314 g·cm−3
Density for aluminum and uncertainty: 2.427 g·cm−3 +/- 0.0463 g·cm−3
Accepted values
Density for lead: 11.34 g·cm−3
Density for iron: 7.874 g·cm−3
Density for aluminum: 2.70 g·cm−3
Conclusion - Comparing both our calculated results and the accepted values we find that both are marginally close. Iron for us was probably the furthest away from the accepted value. Which could either mean the measuring tools were that old or some where along the way we made a mistake numerically. Another reason could be that it may have been a different element. Which we found that the first metal cylinder marked tin may have actually been lead. Overall it would be safe to assume that if all calculation were correct. The calculated values with the range we found in the uncertainty would have the accepted values in each parameter.
Part 2 - Determination of an unknown mass
Purpose - To discover the value of the unknown mass hanging by two angled wires and determining the uncertainty in the calculated values.
These unknown masses are hanging on two wires that are attached to a spring scale. The spring scale will give us a recorded value of tension force. We also have a type of measuring tool that will assist us in obtaining an angle.
We are to pick two unknown masses out of the three and record data. Our data will include; force one, force two, angle one, and angle two. Giving us a total of four variables for each experiment.
Unknown Mass #1 0.7434 kg +/- 0.0949 kg
Unknown Mass #2 0.10255 kg +/- 0.10255 kg
Conclusion - Given the new found ability to propagate uncertainty we were able to give our calculated mass a range of values, if done correctly will yield the accepted value within.









No comments:
Post a Comment